
Just as in the case of the motion of projectiles on earth, the mass of the projectile has no effect upon the acceleration towards the earth and the speed at any instant. The period, speed and acceleration of a satellite are only dependent upon the radius of orbit and the mass of the central body that the satellite is orbiting. None of these three equations has the variable M satellite in them. There is an important concept evident in all three of these equations - the period, speed and the acceleration of an orbiting satellite are not dependent upon the mass of the satellite. Where T is the period of the satellite, R is the average radius of orbit for the satellite (distance from center of central planet), and G is 6.673 x 10 -11 N The period of a satellite ( T) and the mean distance from the central body ( R) are related by the following equation: Since the logic behind the development of the equation has been presented elsewhere, only the equation will be presented here. The final equation that is useful in describing the motion of satellites is Newton's form of Kepler's third law. m 2/kg 2, M central is the mass of the central body about which the satellite orbits, and R is the average radius of orbit for the satellite.Thus, the acceleration of a satellite in circular motion about some central body is given by the following equation In Lesson 3, the equation for the acceleration of gravity was given as g = (G The acceleration value of a satellite is equal to the acceleration of gravity of the satellite at whatever location that it is orbiting. Similar reasoning can be used to determine an equation for the acceleration of our satellite that is expressed in terms of masses and radius of orbit. m 2/kg 2, M central is the mass of the central body about which the satellite orbits, and R is the radius of orbit for the satellite.Taking the square root of each side, leaves the following equation for the velocity of a satellite moving about a central body in circular motion Then both sides of the equation can be multiplied by R, leaving the following equation. Observe that the mass of the satellite is present on both sides of the equation thus it can be canceled by dividing through by M sat.
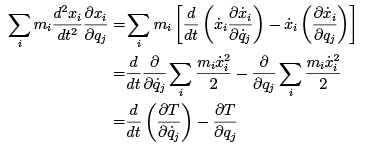
Since F grav = F net, the above expressions for centripetal force and gravitational force can be set equal to each other.
This net centripetal force is the result of the gravitational force that attracts the satellite towards the central body and can be represented as F grav = ( G If the satellite moves in circular motion, then the net centripetal force acting upon this orbiting satellite is given by the relationship F net = ( M sat The central body could be a planet, the sun or some other large mass capable of causing sufficient acceleration on a less massive nearby object. In this part of Lesson 4, we will be concerned with the variety of mathematical equations that describe the motion of satellites.Ĭonsider a satellite with mass M sat orbiting a central body with a mass of mass M Central. The mathematics that describes a satellite's motion is the same mathematics presented for circular motion in Lesson 1. The same simple laws that govern the motion of objects on earth also extend to the heavens to govern the motion of planets, moons, and other satellites. Examples are energy released by fission and fusion.The motion of objects is governed by Newton's laws. Typically this relates to the strong force.

For example, a ball sitting on a table has potential energy with respect to the floor because gravity acts upon it.

It may be considered as energy relating to temperature.


 0 kommentar(er)
0 kommentar(er)
